Maths
WHY WE LOVE MATHS AT WINDMILL

We have a very creative curriculum at Windmill and this doesn’t stop at Maths. Enter into a Maths lesson at Windmill and you will find dancing, singing and children using fun and practical resources.

At Windmill Primary School we aim not only to prepare our children for the next stage of their education, but also to lay the foundations for successful lives after school. A mastery approach to the teaching of mathematics has been adopted, so we have high expectations of all our pupils. What this means is, that we are passionate about all children being supported and challenged, regardless of their ability. We equip children with what they need to succeed in the real world and ensure they are able to apply their skills to real life problems.
The aims of our maths teaching at Windmill are aligned with the aims of the National Curriculum: fluency, reasoning and problem solving. We recognise that pupils need to learn basic number facts and acquire fluency in procedures, alongside developing conceptual understanding if they are to be able to solve increasingly complex problems in life and later in the workplace.
We do not encourage the use of worksheets and instead, encourage children to use a maths book and present their work themselves. We use exit tasks or problems which may be stuck into books, these are to stretch, challenge and enrich the learning. Some children may be scaffolded with some additional things that would be found in books such as number lines or graphs and tables. We never want recording to be a barrier to the children in our school so aim to remove this.
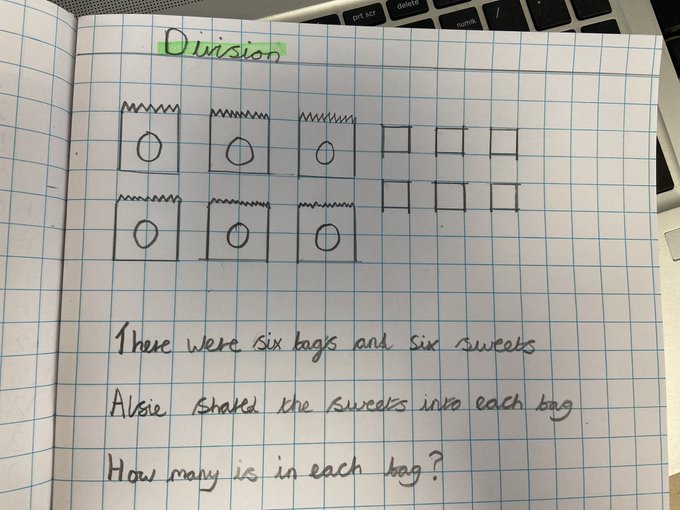
HOW DO WE TEACH MATHS
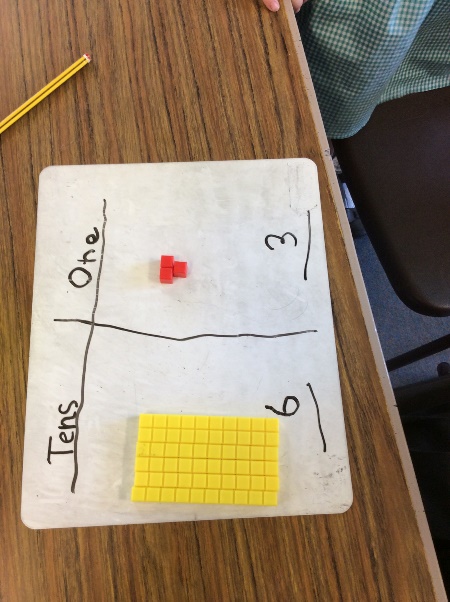
We aim to teach maths in a fun, engaging and interactive style. There is a lot of discussion, working through problems in pairs and children using concrete resources to show their answers. Maths lessons from Year 2-6 begin with a revision of times tables through our times table dance. In EYFS and Year 1, children sing and dance to number songs.
 We have not subscribed to any particular scheme or government recommended text book. Instead, we combine elements of White Rose and some of the best things we see and use our own professional judgement and knowledge of the children to plan our lessons. Lessons follow a clear progression from year to year and from lesson to lesson over units of learning.
We have not subscribed to any particular scheme or government recommended text book. Instead, we combine elements of White Rose and some of the best things we see and use our own professional judgement and knowledge of the children to plan our lessons. Lessons follow a clear progression from year to year and from lesson to lesson over units of learning.
Each of our lessons flow through a number of core parts, and with additional fluency and consolidation and deepening activities, ensure that pupils:
• become fluent in the fundamentals of mathematics, including through varied and frequent practice with increasingly complex problems over time, so that pupils develop conceptual understanding and the ability to recall and apply knowledge rapidly and accurately
• reason mathematically by following a line of enquiry, conjecturing relationships and generalisations, and developing an argument, justification or proof using mathematical language
• can solve problems by applying their mathematics to a variety of routine and non-routine problems with increasing sophistication, including breaking down problems into a series of simpler steps and persevering in seeking solutions
• have a high-quality mathematics education that provides a foundation for understanding the world, the ability to reason mathematically and a sense of enjoyment and curiosity about the subject.
|
‘Maths is fun! We learn about numbers’ EYFS Child |
• meet the expectation that the majority of pupils will move through the programme at broadly the same pace with decisions about when to progress based on the security of pupils’ understanding and their readiness to progress to the next stage. Pupils who grasp concepts rapidly are challenged through being offered rich and sophisticated problems rather than any acceleration through new content. Those who are not sufficiently fluent with earlier material consolidate their understanding, including through additional practice, before moving on. This might be through interventions or by being taken out of the classroom to receive an additional maths input that meets their needs.
METACOGNITION AND GROWTH MINDSET

At Windmill, we base all of our planning on metacognition and how children learn. Because we know that things need to be revisited often in order to create a strong neural pathway, we spend the beginning of each lesson doing recap of taught facts. This is done through Fluent in Five in KS2 and Progression in Addition Facts in KS1.
|
‘I like how Maths challenges me and makes me think’ Year 6 Child |

We do not set for Maths, in lessons or in the classroom, as we believe that children should not have a ceiling placed on their learning and ability. Children at Windmill are encouraged to have a growth mindset and this is deep rooted in our whole school ethos. Children are encouraged to believe they are all capable of learning and doing mathematics, given sufficient time, good teaching, appropriate resources and effort. Making mistakes, using mathematical dialogue and taking part in discussions are important parts of our lessons giving our children the opportunity to reason mathematically, develop an argument, justify decisions and compare the efficiency of different strategies.
